Clearly, h(x) = (mx b)(nx c) is a polynomial of degree 2 and h(x) has two roots The respective roots are when f(x) = 0 and g(x) = 0 This means the graph of h(x) crosses the xaxis at the same two points as f(x) and g(x) Thus, if there are points of tangency then they must occur at these common points on the xaxisComparing Graphs of Functions Work with a partner The graph of f(x) = x is shown Sketch the graph of each function, along with f, on the same set of coordinate axesThe graphs of \(y = f (x)\) and \(y = g(x)\) are said to be translations (or shifts) of the graph of \(y = x^2\text{}\) They are shifted to a different location in the plane but retain the same size and shape as the original graph In general, we have the following principles Vertical Shifts

Create A Graph Of The Combined Function H X F X G X In Which F X X 6 And G X X 6 On Brainly Com
How to find the x value of a function
How to find the x value of a function-To zoom, use the zoom slider To the left zooms in, to the right zooms out When you let go of the slider it goes back to the middle so you can zoom more You can clickanddrag to move the graph around If you just clickandrelease (without moving), then the spot you clicked on will be the new center To reset the zoom to the original clickCalculates a table of the given functions f(x) and g(x) and draws the chart f(x) g(x) range (a, b) partitions n Customer Voice Questionnaire FAQ Chart drawing f(x),g(x) 15 /5 DispNum 1 0454 60 years old level or over / A teacher / A researcher / Useful /



Solved Consider The Following Graphs If F X G X H X Then Find F 3 Show Each Step Clearly With Explanation Course Hero
The x1 you might think shifts the graph to the left but it shifts it to the right So let's just review really quickly what this transformation does y equals half of x xh is a horizontal shift If each is positive it shifts the graph to the right Like when h was one, we had x1 the graph was shifted to the right one unitThe graph of f (x) = x h k contains the points (6, 2) and (0, 2) The graph has a vertex at (h, 5) Describe how to find the value of h Then, explain how this value translates the graph of the parent function Sample Response The absolute value function is symmetric with its vertex on the line of symmetryAngle in standard position versus bearing
Justify your answer List 3 distinct sequences of the Euler path or Euler circuit, starts from Line A ii)Graph of quadratic functions in vertex form g (x) = a (x h) 2 k A quadratic function in vertex form g (x) = a (x h) 2 k is the basic quadratic function f (x) = x 2 that has been transformed 1) From x 2 to (x h) 2 shift h units right if h is positive or h units left if h is negativeSection 36 Transformations of Graphs of Linear Functions 145 EEssential Questionssential Question How does the graph of the linear function f(x) = x compare to the graphs of g(x) = f(x) c and h(x) = f(cx)?
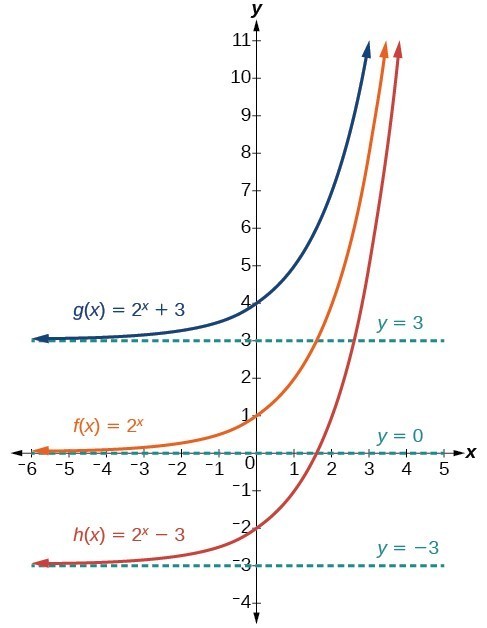
The graph of k(x) is the easiest to identify, since it is the only equation with a growth factor less than one, which will produce a decreasing graph The graph of h(x) can be identified as the only growing exponential function with a vertical intercept at (0,4) The graphs of f(x) and g(x) both have a vertical intercept at (0,2), but since g(x f,g,h and j and we are asked to find which of the following given four graphs represents the graph of the function Clearly when x=0 we see that the function gives the value Hence when x=0 the graph of the function must pass through 10 ie the graph of the function passes through the point (0,10) Hence the the function that represents the graph of the given function is gYes, in 1950 Hellmuth Kneser solved g (g (x)) = e^x on the entire real line with g real analytic You can use the inverse of his solution Cannot imagine there is unicity



Solving Equations Graphically




Write The Functions By The Graphs F X G X H X Brainly Com
Let H (x) f (x)2g (x), where the graphs of f and g are shown in the figure to the right Find H' (5) 10 8 6 XEf (x) 4 2 g (x) 0 2 0 4 6 10 H' (5) cCSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreFind (fºg)(x) and (gºf)(x) F b) B H E Ic A K, i) Does the graph shown above have a Euler path or Euler circuit?



Answered Y 8 8 7 6 5 4 3 2 7 6 Y G X Bartleby



The Graphs Of F X And F X Are Given Below Use The Chegg Com
A function may be thought of as a rule which takes each member x of a set and assigns, or maps it to the same value y known at its image x → Function → y A letter such as f, g or h is often used to stand for a functionThe Function which squares a number and adds on a 3, can be written as f(x) = x 2 5The same notion may also be used to show how a function affects particular valuesPlease Subscribe here, thank you!!! If f is the function whose graph is shown, let h(x) = f(f(x)) and g(x) = f(x^2) Use the graph of f to estimate the value of each derivative, (a) h'(2) (b) g'( 🎉 Announcing Numerade's $26M Series A, led by IDG Capital!



Solved Consider The Following Graphs If F X G X H X Then Find F 3 Show Each Step Clearly With Explanation Course Hero



Shifting Functions Examples Video Khan Academy
H(x) = f (x)g (x) h ( x) = f ( x) g ( x) Since f (x)gx f ( x) g x is constant with respect to f f, the derivative of f (x)gx f ( x) g x with respect to f f is 0 0 0 0A) let f(x) = 4x and g(x) = 2x,x # 0;Answer to Let H(x) = 2f(x) 3g(x), where the graphs off and g are shown in the figure to the right Find H' (1) 10 6 sfox 4 2



Review Problems Integration 1 Find The Instantaneous Rate Of Change Of The Function At X 2 Ppt Download



Q Tbn And9gcq9arl5ab K Kkztqdma6czzlslnfprp6ljv7o6a18 5qqda4yy Usqp Cau
Graph and Formula of f(x) g(x) Discover Resources Algebra Assignment 408;G(x) = (x3)2 = f (x3) h (x) = (x 2)2 = f (x2) Here is a picture of the graph of g(x) = x4 It is obtained from the graph of f(x) = x by shifting it to the right 4 units Horizontal/ Vertical Scaling Horizontal Scaling Let g(x) = f(cx) where c is a positive real numberCreate a graph of the combined function h(x) = f(x)/g(x) in which f(x) = x 6 and g(x) = x 6 On the graph show the graphs of f(x) and g(x) also Answer by ikleyn() (Show Source) You can put this solution on YOUR website!




If F X And G X Are Any Two Differentiable Functions Then Can We Say That Function H X F X G X Is Always Differentiable Quora




The Figures Below Show The Graphs Of The Exponential Functions F X And G X And The Linear Function H X The Function F X Has Y Intercept 0 75 And Goes Through The Point 1 3 2 The
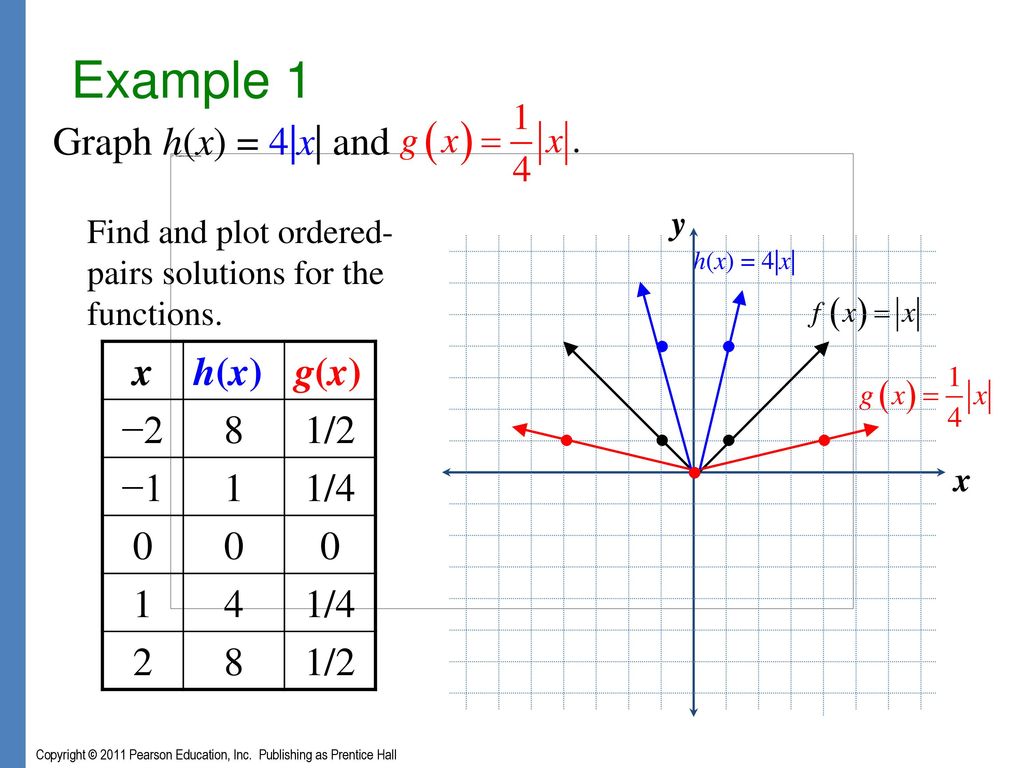
Concavity (new) End Behavior (new) Average Rate of Change (new) Holes (new) Piecewise Functions Continuity (new) Discontinuity (new) Arithmetic & Composition Compositions To start, let's consider the quadratic function y=x 2 Its basic shape is the redcoloured graph as shown Furthermore, notice that there are three similar graphs (bluecoloured) that are transformations of the original g(x)=(x5) 2 Horizontal translation by 5 units to the right;• The graph of f(x)=x2 is a graph that we know how to draw It's drawn on page 59 We can use this graph that we know and the chart above to draw f(x)2, f(x) 2, 2f(x), 1 2f(x), and f(x) Or to write the previous five functions without the name of the function f,




Let H X F X 5g X Where The Graphs Of Fand G Are Shown In The Figure To Homeworklib



The Graph Of A Function F Is Illustrated Mathskey Com
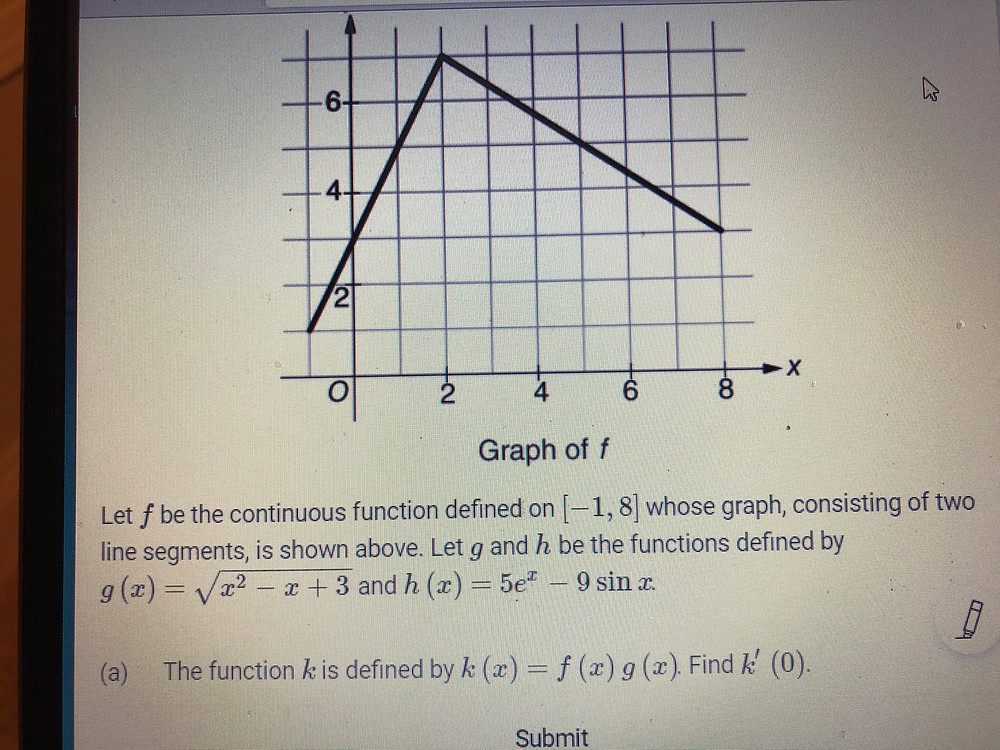
SOLUTION us the graphs of f and g to graph h (x) = (fg) (x) Points for f (3,3), (1,1), (2,2) Points for g (3,1), (1,2), (2,1) I have the sum of the points now at (3,2), (1,1) Could you check my answer I'm thinking you add the y's or something like thatIf so, explain why If not, give a counter example The graph of h(x) = f(x)/g(x) is different than the graphs of this function in the earlier exampleswhy?H′(3) 1 5a A function f (x) has derivative f ′(x) = 3x 18x The graph of f has an xintercept at x = −1 Find f (x) Markscheme evidence of integration (M1) eg correct integration (accept absence of C) (A1)(A1) eg attempt to substitute x = −1 into their f = 0 (must have C) M1 eg



Secure Media Collegeboard Org Digitalservices Pdf Ap Apcentral Ap15 Calculus Ab Q2 Pdf




Using Transformations To Graph Functions
F(x) = f(x) − k Table 251 Example 251 Sketch the graph of g(x) = √x 4 Solution Begin with the basic function defined by f(x) = √x and shift the graph up 4 units Answer Figure 253 A horizontal translation 60 is a rigid transformation that shifts a graph left or right relative to the original graphFirst, the function 5x^22 is performed, and then the result is squared The inside function is performed first, and the outside function is performed second So if f (g (x))= (5x^22)^2, then one possibility could be g (x)=5x^22 for the inside function, and f (x)=x^2 for the outside functionGiven f (x) = 2x, g(x) = x 4, and h(x) = 5 – x 3, find (f g)(2), (h – g)(2), (f × h)(2), and (h / g)(2) This exercise differs from the previous one in that I not only have to do the operations with the functions, but I also have to evaluate at a particular x value



Functions Graphs



2
H x f x c 2 Vertical shifts c units downward h x f x c 3 Horizontal shift c units to the right h x f x c 4 Horizontal shift c units to the left h x f x c Reflections in the Coordinate Axes – Reflections in the coordinate axes of the graph of y = f(x) are represented as follows 1 Reflections in the xaxis h x f x h'(1)=16/3 The product rule states, if h(x)=f(x)g(x), then h'(x)=f'(x)g(x)f(x)g'(x) We are ask to find h'(1), or by the product rule h'(1)=f'(1)g(1)f(1)g'(1) The values of the functions must be f(1)=2 and g(1)=4/3 Remember the derivative gives the slope of any given point, but as we can see in the figures these must correspond, to the slope of the line, whichAngles around a transversal;




Use The Graphs Shown Below And The Function H X 5 2x To Determine The Values Of F H 1 F G 2 F F 3 Study Com




Create A Graph Of The Combined Function H X F X G X In Which F X X 6 And G X X 6 On Brainly Com
For example, consider the functions g (x) = x 2 − 3 and h (x) = x 2 3 Begin by evaluating for some values of the independent variable x Now plot the points and compare the graphs of the functions g and h to the basic graph of f (x) = x 2, which//googl/JQ8NysUse the Graph of f(x) to Graph g(x) = f(x) 3 MyMathlab HomeworkH(x)=x 2 5 Vertical translation by 5 units upwards;



Use The Functions F And G Defined By The Graphs In Fig 10 To Determine The Following Limits A Lim X 2 X H X 1 B Lim X 2 H 3 X 3 X Math
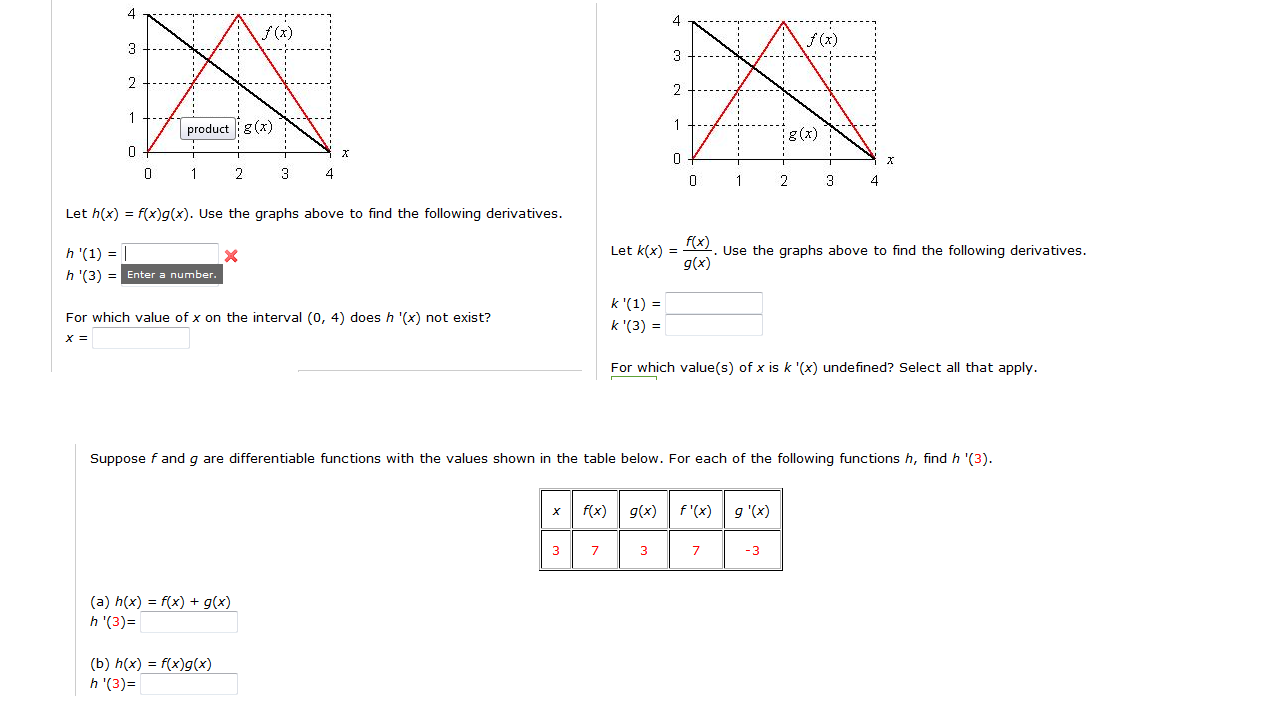



Let H X F X G X Use The Graphs Above To Find The Chegg Com
Let g(x) = f(x) c The graph of g is obtained from the graph of f by shifting up c units Example 1 f(x) = x 2, g(x) = x 2 3 If we subtract c from f(x), then we shift the graph down Let h(x) = f(x) c The graph of h is obtained from the graph of f by shifting down c units Click here for a Toolbook program that illustrates vertical shifts so we have the graphs of two functions here we have the graph y equals f of X and we have the graph y is equal to G of X and what I want to do in this video is evaluate what G of f of F let me do the F of in another color F of negative five is f of negative five is and it can sometimes see a little daunting when you see these composite functions you're taking you're evaluating the function GGraph of the function f(x) = x 4 − 4 x over the interval −2,3 Also shown are the two real roots and the local minimum that are in the interval Definition Given a mapping →, in other words a function together with its domain and codomain , the graph of the mapping is the



Introduction Of Linear Functions



Www Manhassetschools Org Site Handlers Filedownload Ashx Moduleinstanceid 4085 Dataid Filename Aimm 27 with key Pdf
Which transformation of f(x) will produce the same graph as g(x)? First you compute the function for the innermost values That is g ( x 0), then you compute f ( g ( x 0)) and then you multiply by h ( x) I you draw the blue function first, you can use the previous graph to make the next one ( ⌊ x ⌋) = { − 1 if x < 0, 0 if x ∈ 0, 1), 1 if x ≥ 1 ( ⌊ x ⌋) at various values of x25The graph of a function f(x) and its rst and second derivatives are shown below Explain which graph is f(x);f0(x), and f00(x) Solution Graph Arepresents the function f(x) Graph Crepresents the rst derivative function f0(x), and Graph Brepresents the second derivative function f00(x) 12



Business Calculus



2
In this example, f(x) with positive slope and g(x) with negative slope yielded h(x) = f(g(x)) with negative slope Will this always hold?I(x)=(x) 2 Reflection along the origin Given f (x) and g (x) = f (x) k We have to look at the graph and determine the value of k as we can clearly see in the graph that f (0)=2 and g (0)=5 Hence, it must satisfy the equation ie g (0)=f (0)k 5=2k Hence, k=3 bolivianouft and 9




Write The Functions Represented By The Graphs F X G X H X Brainly Com



What Is The Graph Of H X F X G X With An Example Quora
I got this answer by looking at x = –3 on the f(x) graph, finding the corresponding yvalue of 1 on the f(x) graph, and using this answer as my new xvalue on the g(x) graph That is, I looked at x = –3 on the f(x) graph, found that this led to y = 1, went to x = 1 on the g(x) graph, and found that this led to y = –1 SimilarlyThe graph of g(x), the transformed function whose parent function is y=f(x) The graph of y=f(x) is drawn in each of the coordinate systems on the picture graph View transcribed image textIs there a single realvalued function, continuous f 100,∞ → R such that f (f (x)) = logx for every x in its domain?



Which Function Below Has The Smallest Slope F X F X 5x 4 G




Using Transformations To Graph Functions
Free math problem solver answers your algebra, geometry, trigonometry, calculus, and statistics homework questions with stepbystep explanations, just like a math tutorF(x) = 3x g(x) = f(x) 3 A h(x) = f(x) 1 B h(x) = 28x) C h(x) = f(x 1) D h(x) = f(x – 1) Categories Mathematics Leave a Reply Cancel reply Your email address will not be published Comment Name2 Answers So many possible combinations of types of equations for f (x) and g (x) If they are both linear f (x) = 3x 2 g (x) = 2x 5 h (x) = f (x) g (x) = 5x 3 This is also linear f (x) has slope = 3 and yintercept = 2 g (x) has slope = 2 and y intercept = 5 h (x) has slope = 5 and yintercept =




9 8 Graph Quadratic Functions Using Transformations Mathematics Libretexts



Lines Slope And Functions



Search Q Derivative Graph Tbm Isch
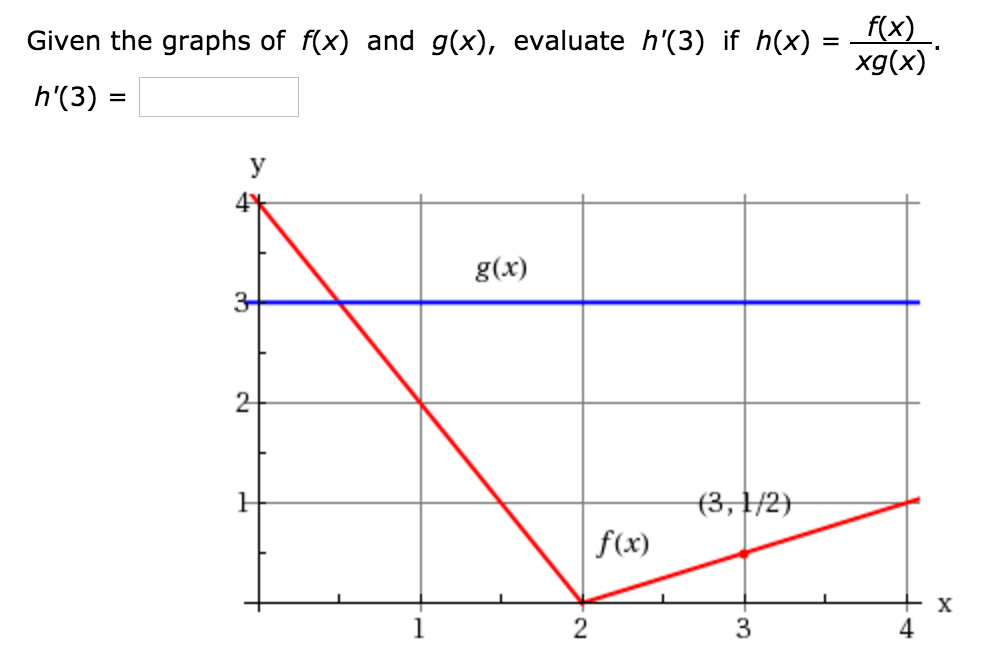



Given The Graphs Of F X And G X Evaluate H 3 If Chegg Com




Answered 71 Let R X B If H X G F X Fnd Bartleby



2



F X G X H X 1
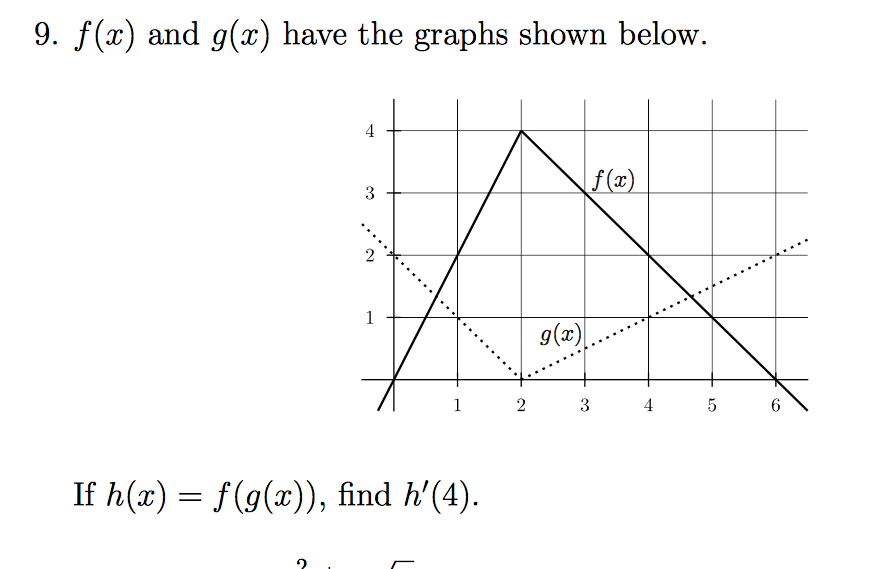



F X And G X Have The Graphs Shown Below If H X Chegg Com



Faculty Atu Edu Mfinan 13 Lecture6 Pdf




Function Of The Form Max F X G X H X



Solution I Am Struggling So Much With Trying To Do This Please Help Create A Graph Of The Combined Function H X F X G X In Which F X X 6 And G X



Section 1 5 Combinations Of Functions Ppt Download




View Question Translating Units In A Graph



Www Muncysd Org Cms Lib Pa Centricity Domain 104 ap calculus practice exam by patrick cox Pdf



2



The Graph Of The F X Is Show Below Graph Each Transformed Function And List In Words The Transformation Used Socratic



Solution Draw The Graph Of F X X If G X F X 5 If H X F X 6 How Is The Graph F X Translated To Form The Graph G X How Is The



Www Southhadleyschools Org Cms Lib Ma Centricity Domain 11 Frq solutions Pdf



Secure Media Collegeboard Org Digitalservices Pdf Ap Apcentral Ap15 Calculus Ab Q2 Pdf




Let F X Be As Displayed In The Graph Below Estimate H 2 G 2 And J 2 Where H X F F X G X F X 2 And F X Sqrt F X If An Answer Does Not Exist Indicate With Dne



Www Math Fsu Edu Bellenot Class F00 Cal1 Test1 Pdf




Exponential Functions Ck 12 Foundation



Horizontal Stretch Properties Graph Examples



Http Smacmathapcalculus Weebly Com Uploads 1 9 2 5 Apc 3 6 Hw Key Pdf




Graphing Functions With Excel




The Graphs Below Are Of The Functions F G And H Defined By F X Log 4 X G X Log 5 X And H X Log 6 X Snapsolve
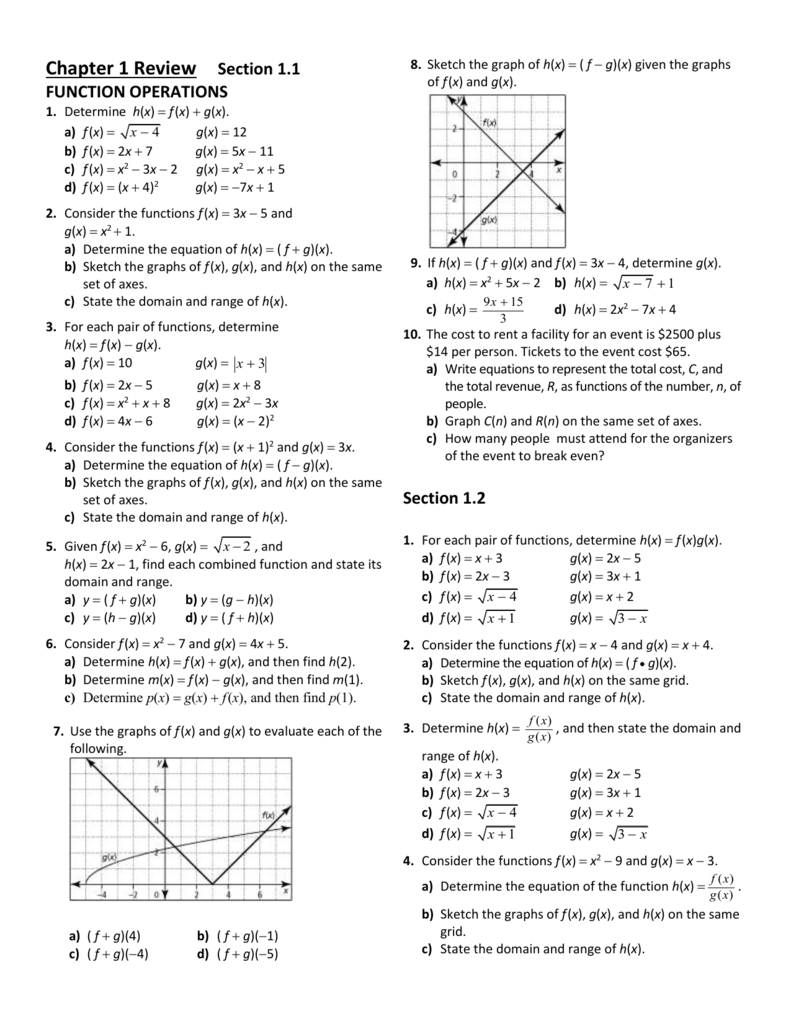



Chp 1 Function Operations Review



Http Www Opentextbookstore Com Precalc 2 Precalc1 5 Pdf




Precalculus Shifting Reflecting And Stretching Graphs Flashcards Quizlet



Graph Exponential Functions Using Transformations College Algebra



Let H X F X G X And K X F X G X Use The Figures Below To Find The Exact Values Of The Indicated Derivatives H 1 And K 1 Socratic




Grade 12 Pre Calculus Composition Of Functions How Do I Graph G X F X Homeworkhelp



F X F X F X Relationship



What Is A Function Transformation Expii



Chapter 3 Graphs And Functions Ppt Download



I Need Help Finding G X Bothell Stem Coach



2 2e Exercises Mathematics Libretexts




Help Needed With Calculus Question Wyzant Ask An Expert




Here Are Graphs Of Two Functions F And G The Function H Is Defined By H X F X G X 1 For Which Brainly Com



2



Graph Quadratic Functions Using Transformations Intermediate Algebra



The Functions F And G Are Shown In The Figure Above If H X F G X Youtube
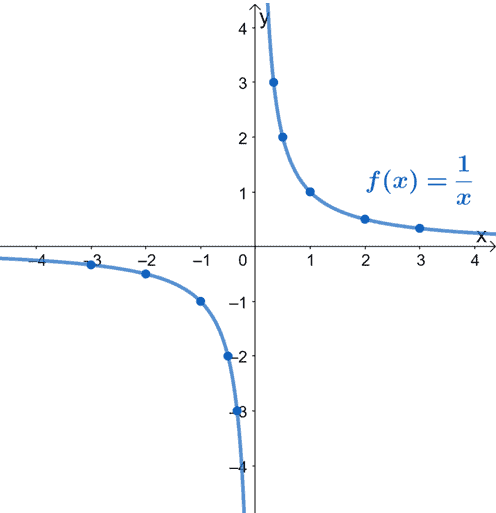



Reciprocal Function Properties Graph And Examples



2
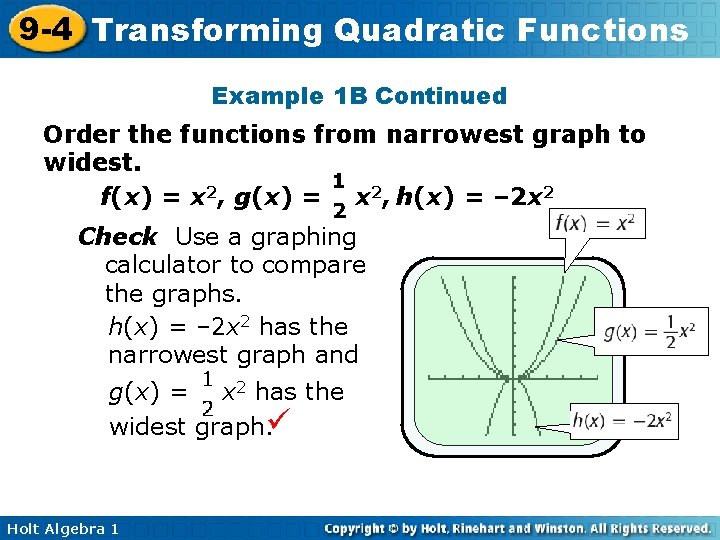



9 4 Transforming Quadratic Functions Objective Graph And



2




The Figure Above Shows The Graphs Of The Functions Chegg Com
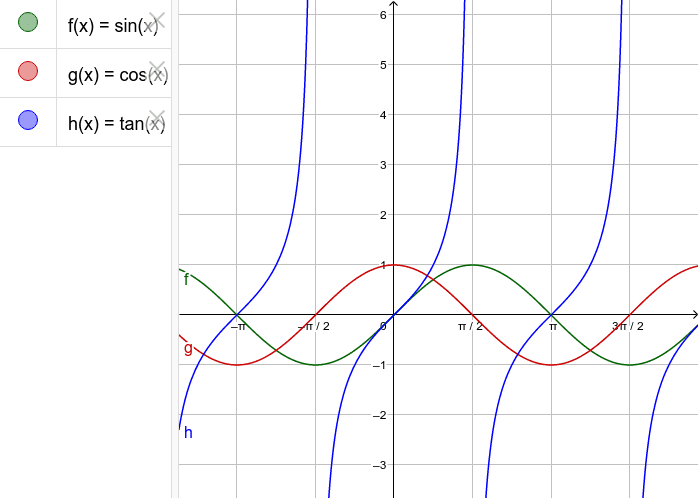



The Functions Sin X Cos X And Tan X Geogebra
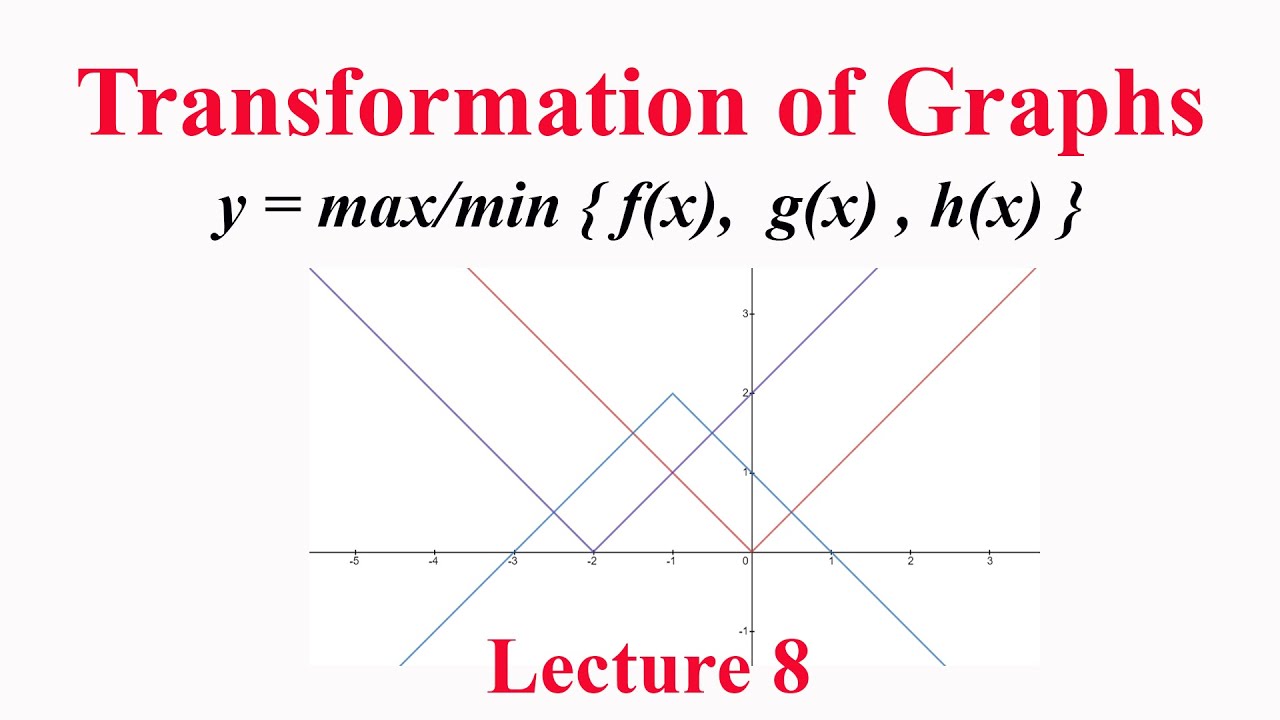



Graphs Of Functions Defined As Y Max Min F X G X H X Transformation Of Graphs Lecture 8 Youtube
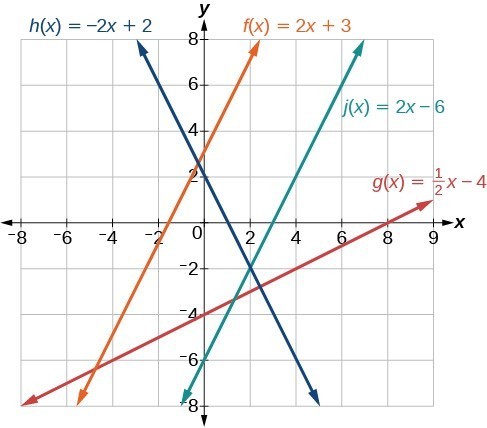



Parallel And Perpendicular Lines College Algebra



Http Math Arizona Edu Cjewell 122b Handouts Derivativepractice Pdf



Solved Sy G X X The Graphs Of F And G Are Shown Above Course Hero



Untitled Document
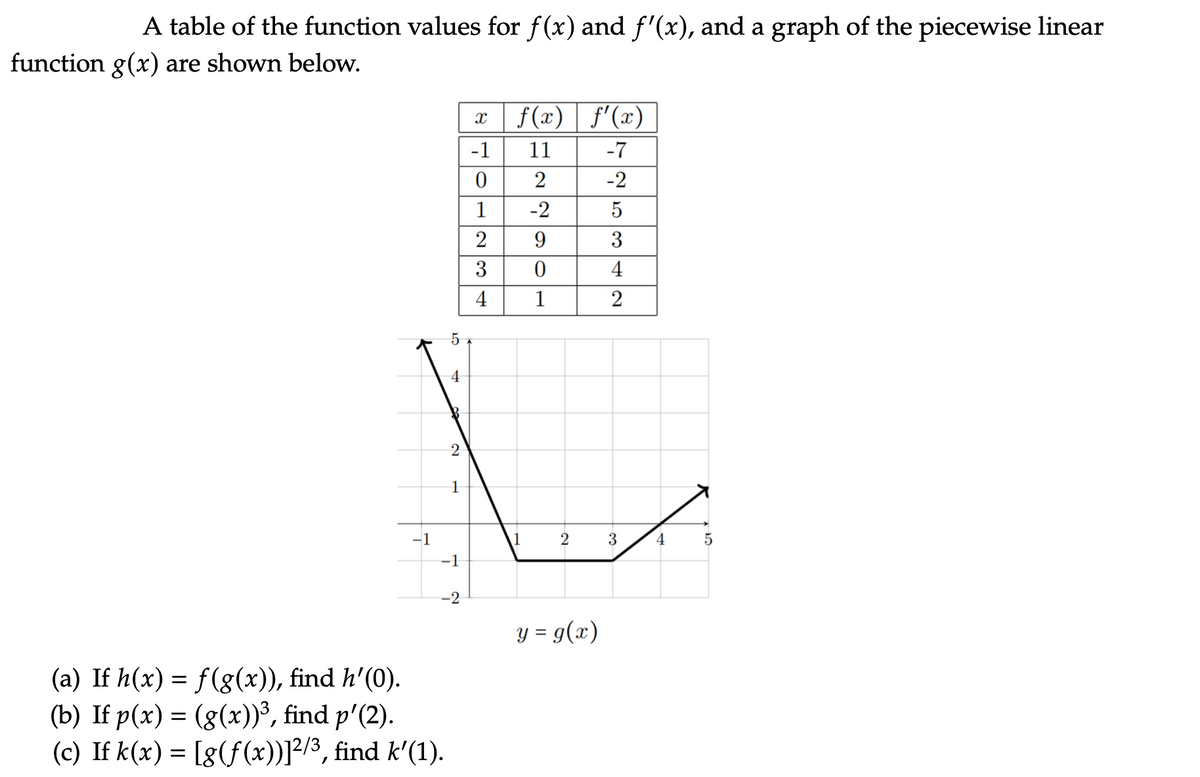



Answered A Table Of The Function Values For F X Bartleby



F X G X H X 1



F X G X H X 1




Consider The Following Graphs If F X Frac G X H X Then Find F 3 Show Each Step Clearly With Explanation Study Com




Graphing Functions With Excel



Http Www Pearlandisd Org Cms Lib Tx Centricity Domain 2956 Notes for 4 3 Pdf



Http Www Math Tamu Edu Bollingr 131wir 131ctk2 Pdf



Search Q Composite Functions Tbm Isch
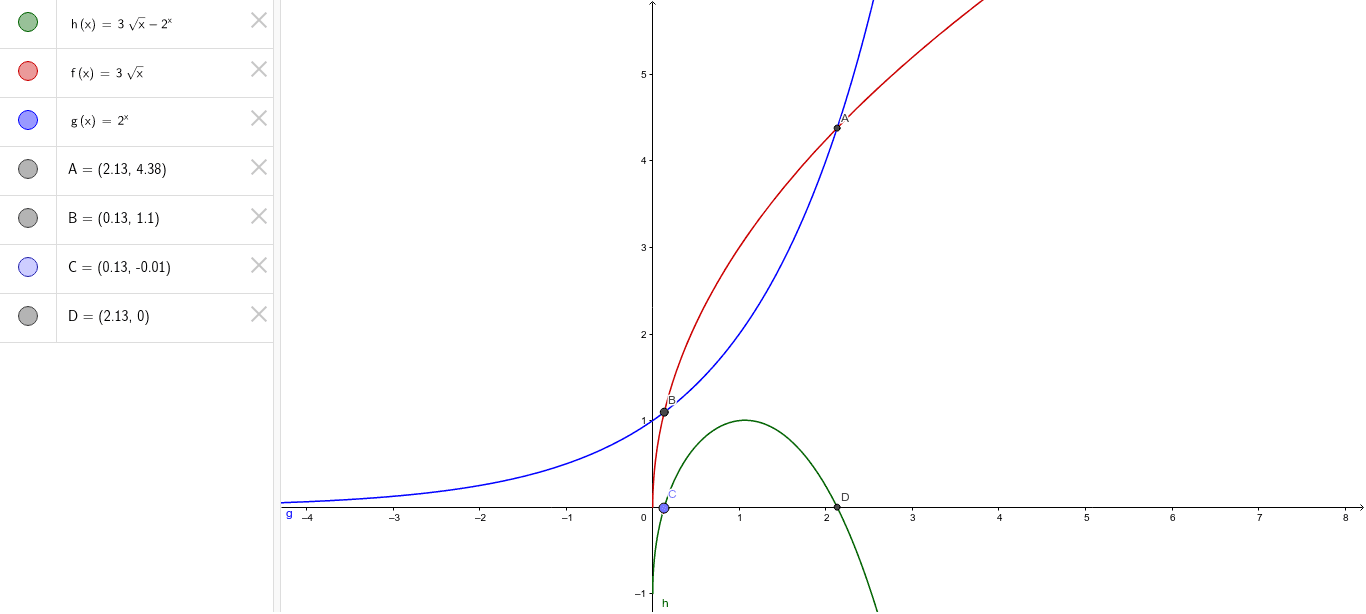



Graph Of F X G X F G X Geogebra
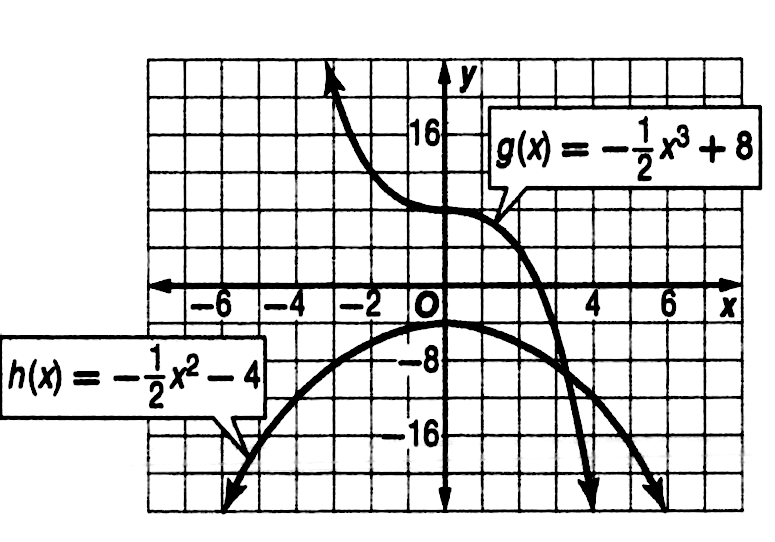



In Each Problem The Graphs Of G X And H X Are Shown Use Addition Of Ordinates To Graph A New Polynomial Function F X Such That F X G X H X Then Write The Equation For F X
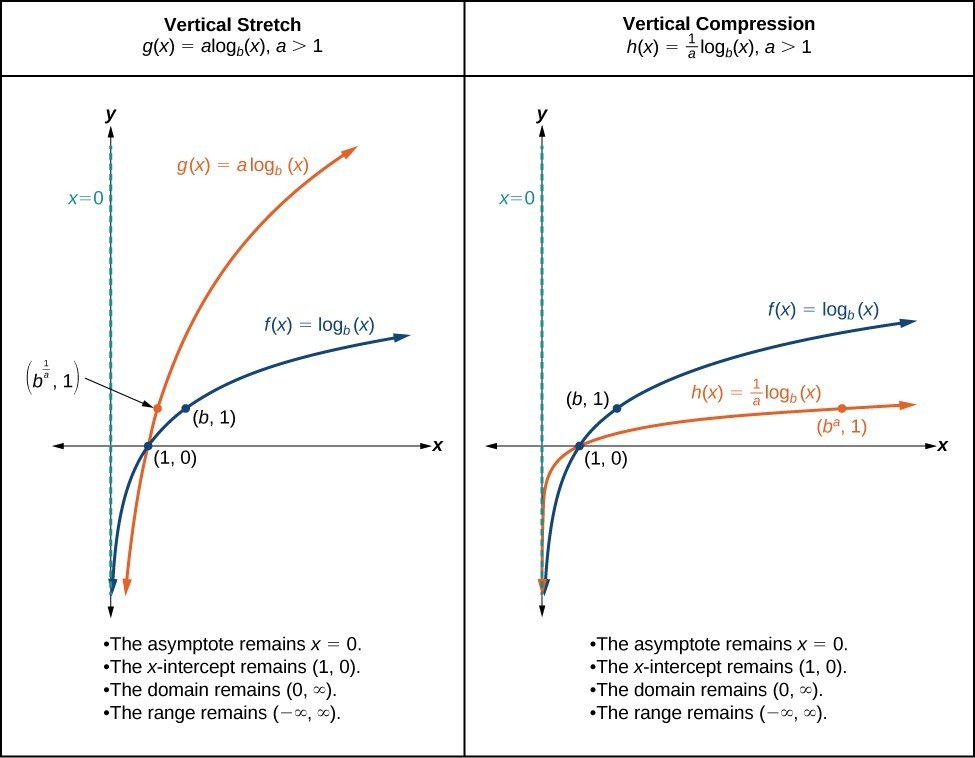



Stretching Compressing Or Reflecting A Logarithmic Function College Algebra



New Functions From Old



2




Quotient Rule Wikipedia




Function Notation




Using The Graphs Below Select The Graph That Represents H X Given That Function H X F X G X




Name Group Members Exploration 4 2a Derivative Of A Pages 1 12 Flip Pdf Download Fliphtml5



F X G X H X 1



2




Solved If F Is The Function Whose Graph Is Shown Let H X F F X And G X F X 2 Use The Graph Of F To Estimate




5 Given Below Are The Graphs Of Two Functions X And G X Both Functions Are Defined On 0 5 Let H X G X Use The Graphs To Determine The Sevalues Of The Critical Points Ofh X Explain Credit W



Get Answer Three Different Functions F X G X And H X Have The Same Transtutors
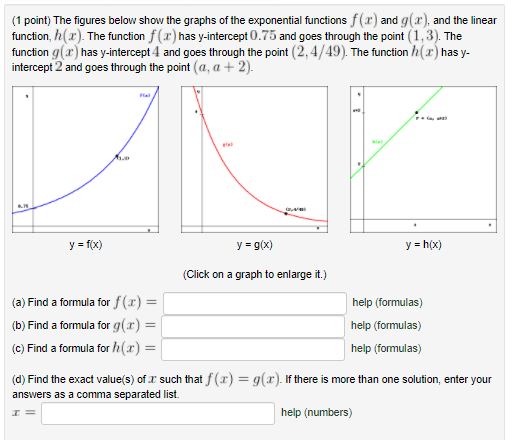



1 Point The Figures Below Show The Graphs Of The Exponential Functions F X And G X And The Linear Func



0 件のコメント:
コメントを投稿